Perinatal mortality in Belarus

The region (oblast) of Gomel is the area with highest fallout in Belarus. Whereas little strontium in the Chernobyl fallout was measured in Munich (210 Bq/m² Sr-90 compared to about 20,000 Bq/m² Cs-137, May 1986), the strontium soil contamination in parts of Gomel oblast outside the 30-km exclusion zone exceeded 37,000 Bq/m² in 1986.

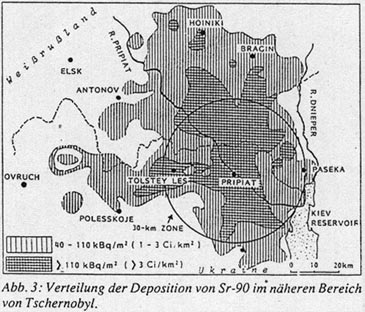
Strontium-90 soil contamination near the Chernobyl reactor site (from German journal Atomwirtschaft, March 1991). The hatched areas indicate strontium concentrations greater than 1 Ci/km² (37 kBq/m²) and 3 Ci/km² (111 kBq/m²), respectively. The circle is the 30-km evacuation zone.

The trend of perinatal mortality rates, 1985-1998, in the oblasts of Gomel, Minsk-City, and in Belarus minus Gomel and Minsk-City are displayed in the next figure. In 1994 there is a 20% increase of perinatal mortality in all three data sets which is a consequence of a change of definition  of stillbirths. Perinatal mortality data from the City of Minsk do not conform with the data in the rest of Belarus. The rates from Minsk-City are consistently higher than in the rest of Belarus until after 1995 when they suddenly drop by 50%. No such decrease occurs in the other regions of Belarus, i.e. the peculiarity in the City of Minsk, the capital of Belarus, is not likely to have biological reasons. Therefore the data for the City of Minsk were not included ih the control area (Belarus minus Gomel and the City of Minsk).

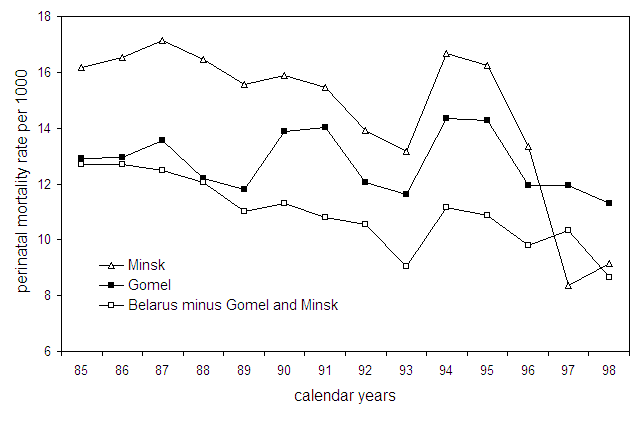
Perinatal mortality rates in Gomel, Minsk-City, and Belarus minus Gomel and Minsk-City. The offset in 1994 results from a definiton change of stillbirths.

A trend analysis of perinatal mortality data is problematic for two reasons. First - like in most European countries - the definition of stillbirth was changed at the end of 1993. Second, possible socio-economic problems after the break-up of the Soviet Union in 1991 might have had an influence on the trend of perinatal mortality rates. Assuming that these influences acted equally in the study and the control region, a possible effect of the radiation exposure should be found in the ratio of perinatal mortality rates in Gomel oblast to the rates in the control area.

Method

The ratio of perinatal mortality rate p₁ in Gomel to the rate p₀ in the control area can be expressed by the odds ratio (OR) which is defined by

OR = (p₁/(1-p₁)) / (p₀/(1-p₀)).

A weighted regression of the logarithms of the odds ratios is performed with the model

(5.1)    ln(OR) = β₀ +β₁·d₈₇ +β₂·sr(t)

where parameter β₀ allows for a difference in the base line perinatal mortality rates in the study and the control area, β₁ estimates the caesium effect in 1987 (dummy variable d₈₇) and β₂ estimates the possible effect of strontium on the data.

The expression sr(t) is the average strontium concentration in pregnant women, calculated under the assumption that strontium is mainly incorporated at the end of the period of menarche, the time of maximum bone growth, at about age 14. For a given year following Chernobyl, the average strontium concentration is approximated by the percentage of pregnant women who were 14 years old in 1986, i.e. who were born in 1972. This percentage follows from the maternal age distribution. In Belarus, maternal age distributions were only available in 5 year strata (see figure below). The shaded areas represent the average age distribution in Belarus for 1992-1996. To get approximated yearly values, a superposition of two lognormal distributions was used to fit the step function.

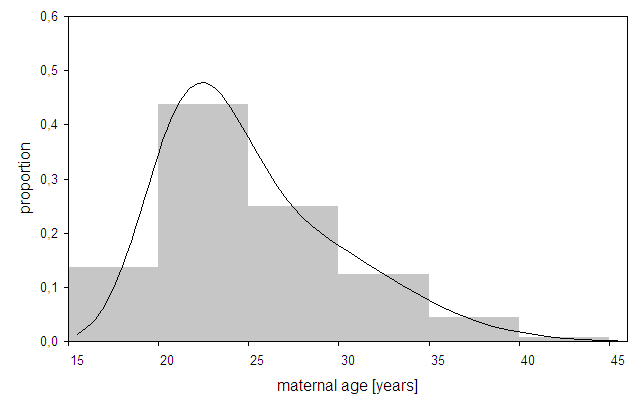
Maternal age distribution in Belarus, averaged for 1992-1996, and interpolation curve using two superimposed lognormal distributions.

Second, the strontium excretion from the body must be considered in the calculation of sr(t). According to the ICRP Publication 67, the strontium excretion cannot be described by a simple exponential decrease, but is composed of a fast and a slow component. Thus, the strontium term sr(t) in year t after Chernobyl has the form

sr(t) ~ F(t-1972)·(A₁·exp(-ln(2)·(t-1986)/T₁)+A₂·exp(-ln(2)·(t-1986)/T₂))

where F(t-1972) is the fraction of pregnant women born in 1972. T₁=2.4 years and T₂=13.7 years are effective half-lives of strontium in the female body. The constants A₁, A₂ and the half-lives T₁, T₂ were determined from a regression of tabulated values given in ICRP publication 67.

For the regression the data are population weighted with weights

var = 1/n₁ + 1/(N₁-n₁) + 1/n₀ + 1/(N₀-n₀)

where n₁ and n₀ are the number of perinatal deaths in the study(1) and control(0) area and N₁, N₀ the corresponding numbers of live births.

To test the significance of the parameters, two-sided t-test are applied (H₀: β₁=0, β₂=0).

Results

To determine the age of maximum strontium uptake from the data, regressions with strontium terms for maximum strontium uptake at age 13, 14, and 15 are performed. The results for the sums of squares are 9.7, 7.3 and 9.9, respectively. Thus the strontium term with an age of 14 years for maximum strontium uptake fits the data best.

The regression yields β₀ = 0.022 ± 0.027, i.e., there is no appreciable difference in perinatal mortality rates between study and control area before Chernobyl. The increase in 1987 is not significant (β₁=0.055 ± 0.060).

To determine the significance of the strontium effect, the difference of the sum of squares resulting from regressions without and with the strontium term is used. The corresponding sum of squares are 29.7 (df=12) and 7.3 (df=10). The corresponding F-test is significant (p=0.0028, F-test).

The figure below shows the observed (dots) and expected odds ratios (solid line) resulting from a regression with β₀=0 (see equation 5.1). In the beginning of the 1990’s, the odds ratios were around 1.3, i.e., perinatal mortality rates in Gomel were 30% higher than in the control area. The excess perinatal mortality rates in Gomel translate to 430 excess perinatal deaths, 1987-1998.

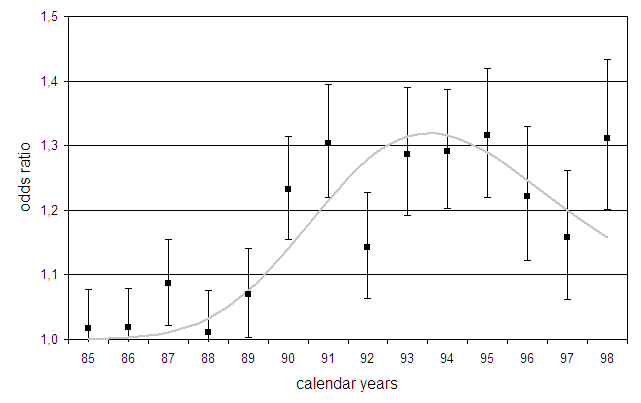
Odds ratios of perinatal mortality in Gomel vs. Belarus minus Gomel and Minsk-City, and regression line.